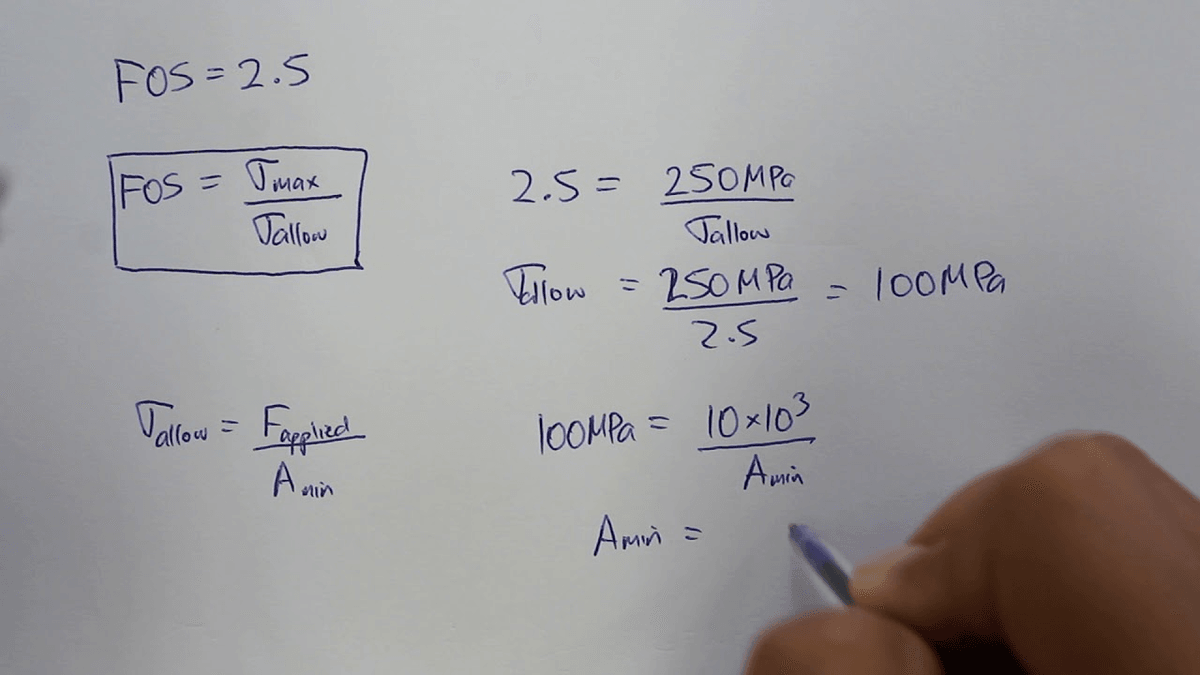
Where x c,i and y c,i are the rectangular coordinates of the centroidal location of the i th section with respect to the reference point, and A i is the area of the i th section. If a cross section is composed of a collection of basic shapes whose centroidal locations are known with respect to some reference point, then the centroidal location of the composite cross section can be calculated as: The centroidal locations of common cross sections are well documented, so it is typically not necessary to calculate the location with the equations above.
Where dA represents the area of an infinitesimally small element, A is the total area of the cross section, and x and y are the coordinates of element dA with respect to the axis of interest. If the exact location of the centroid cannot be determined by inspection, it can be calculated by: If the area is symmetric about only one axis, then the centroid lies somewhere along that axis (the other coordinate will need to be calculated). If the area is doubly symmetric about two orthogonal axes, the centroid lies at the intersection of those axes. The centroid of a shape represents the point about which the area of the section is evenly distributed. Structural Calculators Properties of Areas Centroid.